Standard Deviation Calculator
This free online standard deviation calculator computes the standard deviation, variance, mean, sum, and error margin of a given data set. Enter your numbers below, the Mean, Vraiance and Standard Deviation are calculated live....
If you use this great tool then please comment and/or like this page.
Average Rating: Tool Views: 359
Average Rating: Tool Views: 359
Subscribe for Latest Tools
How to use this Standard Deviation Calculator Tool?
How to use Yttags's Standard Deviation Calculator?
- Step 1: Select the Tool
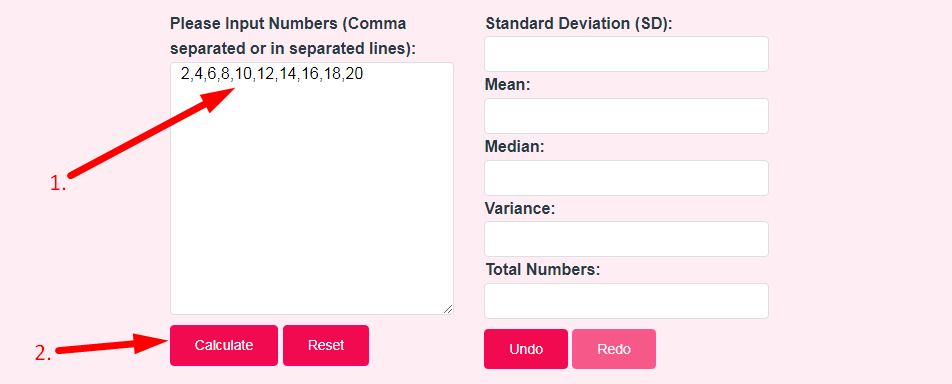
- Step 2: Please Input Numbers (Comma separated or in separated lines) And Click On Calculate Button.
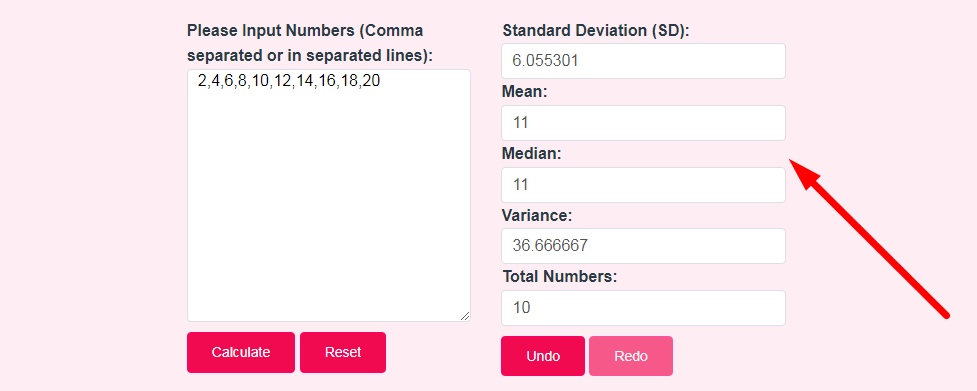
- Step 3: Check Your Standard Deviation Calculator Result
Calculates standard deviation and variance for a data set. Calculator finds standard deviation, the measure of data dispersion, and shows the work for the calculation.
If you want to link to Standard Deviation Calculator page, please use the codes provided below!

FAQs for Standard Deviation Calculator
What is a Standard Deviation Calculator?
A Standard Deviation Calculator is a statistical tool used to calculate the standard deviation of a dataset. It quantifies the amount of variation or dispersion in the data points from the dataset's mean, providing insights into data distribution and variability.
What are the rules for calculating standard deviation?
In this method, we first compute the mean of the data values (¯x x ¯ ) and then compute the deviations of each data value from the mean. Then we use the following standard deviation formula by actual mean method: σ = √(∑(x−¯x) ( x − x ¯ ) 2 /n), where n = total number of observations.
How is a standard deviation calculation useful?
Standard deviation is important because it helps in understanding the measurements when the data is distributed. The more the data is distributed, the greater will be the standard deviation of that data.
Is standard deviation the range?
Range, variance, and standard deviation all measure the spread or variability of a data set in different ways. The range is easy to calculate—it's the difference between the largest and smallest data points in a set. Standard deviation is the square root of the variance.
Can standard deviation be negative?
No, standard deviations cannot be negative. They measure the variation in a dataset, calculated as the square root of the variance. Since variance, a mean of squared differences from the mean is always non-negative, the standard deviation, being its square root, cannot be negative either.